VolumeRendering
images by Gordon Kindleman and Joe Kniss
Multi-Dimensional Transfer Functions
← Gradient-Magnitude And MR | ● | Spatialized Transfer Functions →
In principle a transfer function can have arbitrary dimensions, for example it can depend on the scalar value $s$, the gradient magnitude $g$ and curvature $c$. Hence it would be a 3D function TF(s,g,c).
TF with more than a single dimension are called multi-dimensional transfer functions (introduced by Gordon Kindleman).
G. Kindlman und J. Kniss: Pick a volume and show the respective voxel properties in a multi-dimensional histogram depending on the scalar value and the gradient magnitude:
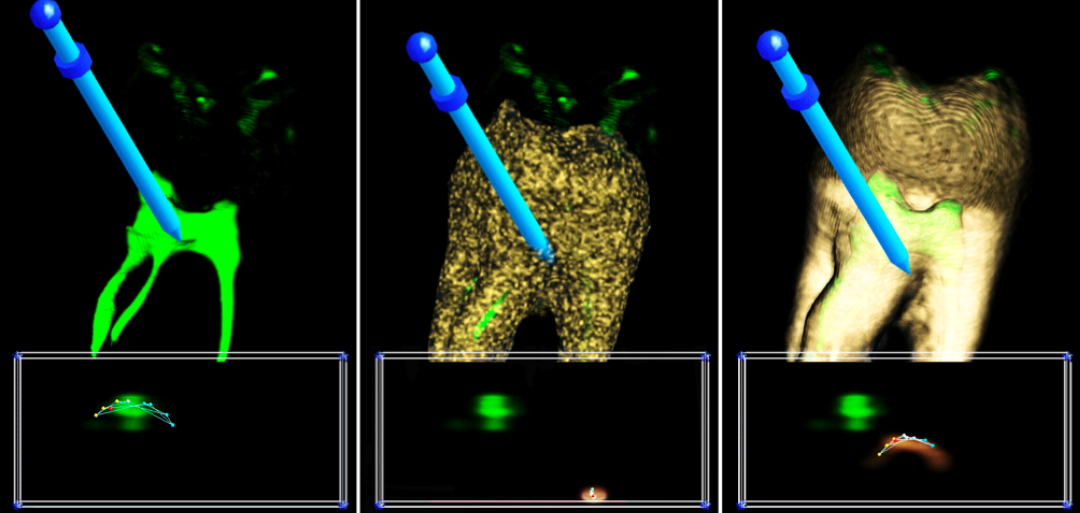images by Gordon Kindleman and Joe Kniss
← Gradient-Magnitude And MR | ● | Spatialized Transfer Functions →